CPhill

At first, Adam had A dollars and  Ben had 600 - A dollars

A 

B = 600 - A

After  Adam gave (1/4) of his  money to  Ben

Adam had  (3/4)A

Ben had   600 - A + (1/4) A  =  600 - (3/4)A

After Ben gave (1/3)  of his  money to Adam

Adam had  (3/4)A +  [ 600 - (3/4)A ] / 3  =    200 + (1/2)A

Ben had   (2/3) [ 600 - (3/4)A ]  =  400 - (1/2)A

And in the end  Ben had (3/2)as much as Adam

400 - (1/2)A  =  (3/2) (200 + (1/2)A )

400 - (1/2)A  =  300 + (3/4)A

100 =  (5/4)A

100 (4/5) =  A  =  $80   (Adam  started with $80 )

Proof :

At first   :   A = 80      B =  520

After the first transaction :     A = 60      B =  540

After the second transacyion :   A = 240  B = 360   ⇒   B/ A = 360 / 240  = 3 / 2

The y intercept  = 0

See here :

From the center of the  small circle  draw a perpendicular to  the bottom  edge of the  square

  .....the distance = 4-r   = A

From  the  center of the semi-circle draw a segment to the point where the first segment intersects the base

.....this distance is 2-r  = B

Connect the center of the  small circle to the center of the semi-circle....the distance is 2 + r = C

We have a right triangle  such that

C^2 = A^2 + B^2

(2 + r)^2  = (4- r)^2  + (2-r)^2    simplify

r^2 + 4r + 4 =  r^2 -8r + 16  + r^2 - 4r + 4

r^2 + 4r + 4  =  2r^2 - 12r + 20

r^2  - 16r + 16  =  0

r^2 -16r   =  -16

r^2 -16r  + 64   = -16 + 64

(r - 8)^2  =  48

r - 8  =   sqrt (48)           or      r - 8 = -sqrt (48)

The first value for r is too large

The second value produces   r =  8 -sqrt (48)  ≈  1.07

\(f(x) = \left\lfloor\frac{2 - 3x}{3x + 1}\right\rfloor \)

Write this  in another way

floor  [  (-3x + 2) / (3x + 1) ] 

Evaluating this  function (without the floor) at f(1)  = -1/4    and floor (-1/4)  = -1

And any positive integer  value of  x produces a function value (without the floor)   which is greater than -1  but less than 0  

So the floor of all such values =  -1

So

f(1)  + f(2)  +......+ f(999) + f(1000)   =

1000 (-1)  =   -1000

{ 11, 13 , 17, 19, 23 ,29, 31}

11 *  13  = 143                 13 * 17 = 221                     17 *  19 =  323

11 *  17  = 187                 13 * 19 = 247                     17 *  23  =  391

11 *  19  = 209                13 *  23 = 299

11 *  23  = 253                 13 * 29 = 377

11 *  29 =  319

11 *  31 =  341

12 different products

Total number  of ways to get from (0,0) to (6,7)  less the number of ways to get from (0,0) to ( 2,3)

C(13,6)  - C(5,2)  =   1716 -10  =  1706

Let BC  = x

Triangles AEC  and BDC  are similar

BC / BD  = AC / AE

x / 1 = (4 + x) / 3

3x = 4 + x

2x = 4

x = 2 =  BC

{ DC = sqrt (BC^2 - BD^2)  = sqrt ( 2^2 - 1^2)  = sqrt (3)

tan(angle DCB)  =  - ( BD / DC)  = -1/sqrt (3)

Equation of tangent line       : y = (-1/sqrt 3) ( x -6)   }

Here :

https://web2.0calc.com/questions/counting-problem_20562

We can find the  x  coordinate of A thusly

12^2   - x^2  = 17^2 - (18 - x)^2

144 - x^2  = 289 - (x^2 - 36x + 324)

144 = -35 + 36x

179 = 36x

x = 179 / 36

And we can find the y coordinate of A as follows

sqrt [ 12^2 - (179/36)^2 ] =  sqrt  [154583 ] / 36

So  A =  ( 179/36, sqrt [ 154583] / 36 )

Let the midpoint of  BC  = (9,0)  = D

AG will be 2/3 of the distance AD

So

AG  =  (2/3)sqrt  [ ( 179/36 - 9)^2  +  (154583/36^2) ]  = sqrt [ 542] / 3   ≈ 7.76

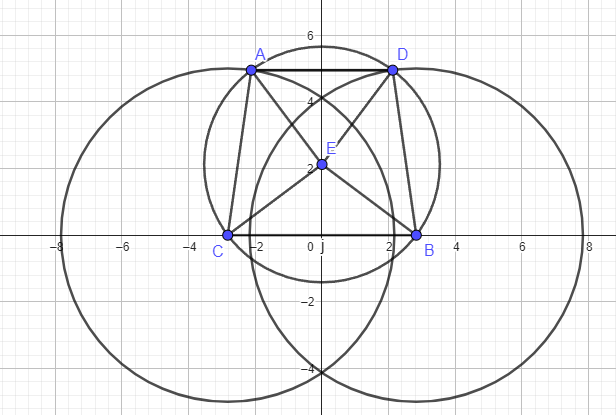
We can construct a trapezoid  with bases

CB = sqrt (32) =  4sqrt (2)

And  AD = sqrt (18) = 3 sqrt (2)

Let C = (-4sqrt (2) / 2 , 0) =  (-2sqrt (2),0)  = (-sqrt (8) , 0)

And by symmetry,  B =(sqrt (8) , 0)

Note that DB =   sqrt ( 3^2 + 4^2)  = 5

Construct circles  with radius = 5  at C , B

The equation for the circle at B =   (x -sqrt 8)^2 + y^2   = 25 

D will have the coordinates   ( 3sqrt (2)/2 , y)  =  (3/sqrt (2)  ,y)

And by symmetry A = ( -3/sqrt (2) , y)

We can  find the y coordinate for D   by solving this  for  y

(3/sqrt2 - sqrt 8)^2  + y^2  = 25     ⇒   y =  7/sqrt 2

So D = (3/sqrt (2) , 7/sqrt (2) )

The center of the circle through ABCD    =  E = (0, y)

We can find y by equating  the distance  from E to B  and  from E to D

So

(sqrt (8) - 0)^2 + y^2 =  ( 3/sqrt 2 - 0)^2 + (y - 7/sqrt 2)^2  

Solving this for  y  produces  3/sqrt (2)

So  E = (0, 3/sqrt (2))

The radius of the circle  can  be  found  as EB =   sqrt [  (sqrt (8) - 0)^2  + (3/sqrt (2) - 0)^2 ] =

sqrt [ 8  + 9/2]  = sqrt [ 25 / 2 ]  =   5 / sqrt (2)

So....the diameter  =  2 * 5 / sqrt (2) =  5 (2 / sqrt (2)  = 5sqrt (2)   { as Holtran found !!! }