Do you know what y=cos(x) looks like?
Graph it on desmos if you do not know..
What is its period?
Where does it first cross the x axis?
https://www.desmos.com/calculator/xbk6uv697u
Try drawing cos(12x) how does it compare?
Try drawing them, discuss it here, if you have specific questions then ask with a new post on this thread.
| 1-z | |1-z|^2 | |||
| 1--1 = 2 | 4 | |||
| \([1+cos(\frac{2\pi}{7})]+isin(\frac{2\pi}{7})\) | \(1+2cos(\frac{2\pi}{7})\) | |||
| \([1-cos(\frac{3\pi}{7})]+isin(\frac{3\pi}{7})\) | \(1-2cos(\frac{3\pi}{7})\) | |||
| \([1-cos(\frac{\pi}{7})]+isin(\frac{\pi}{7})\) | \(1-2cos(\frac{\pi}{7})\) | |||
| \([1-cos(\frac{\pi}{7})]-isin(\frac{\pi}{7})\) | \(1-2cos(\frac{\pi}{7})\) | |||
| \([1-cos(\frac{3\pi}{7})]-isin(\frac{3\pi}{7})\) | \(1-2cos(\frac{3\pi}{7})\) | |||
| \([1+cos(\frac{2\pi}{7})]-isin(\frac{2\pi}{7})\) | \(1+2cos(\frac{2\pi}{7})\) | |||
\(\displaystyle sum = \frac{1}{4}+\frac{2}{1+2cos\frac{2\pi}{7}}+\frac{2}{1-2cos\frac{3\pi}{7}}+\frac{2}{1-2cos\frac{\pi}{7}}\\ \\~\\\displaystyle sum \approx 8.3478346790362325\)
2/(1+2cos(2pi/7)) = 0.8900837358250465
2/(1-2cos(3pi/7)) = 3.603875471605593
2/(1-2cos(pi/7) = -2.493959207437541
0.25+0.8900837358250465+3.603875471605593+3.603875471605593 = 8.3478346790362325
Thanks Geno :)
Attn Gamemaster.
This question is almost identical to one you asked a day of so ago.
https://web2.0calc.com/questions/given-the-graph-of-y-f-x-below-answer-all-of-the
If you do not understand you should say so.
You should always give a written response anyway!
We are not here so that you can copy our answers and learn nothing.
If you did know how to do some but not all then you should have discussed what you already knew!
Continued,
the end of my table got truncated :/
The entries were
| 1/|1+z^2| | |
| 0 | undefined. Can't divide by 0 |
| \(1-2cos(\frac{2\pi}{7})\) | |
| \(1+2cos(\frac{3\pi}{7})\) | |
| \(1+2cos(\frac{\pi}{7})\) | |
| \(1+2cos(\frac{\pi}{7})\) | |
| \(1+2cos(\frac{3\pi}{7})\) | |
| \(1-2cos(\frac{2\pi}{7})\) |
So after all that unnecessary work.
I get that the sum is undefined becasue the first term in the sum is undefined.
Please give feed back on whether the answer is simply undefined. OR on how I should have done it.
Thanks.
This is a reapeat question. You should have sent us with a link, to the original.
Never mind, I do not think you got any answers there.
I played with this for quite a while when you first posted it.
I rarely know the short cuts for complex numbes.
All I could thinkg of doing was getting the 7 answers and adding them up.
If it hadn't been over 1 this would not have been so bad but being over 1 definitely added to the messiness and length of it.
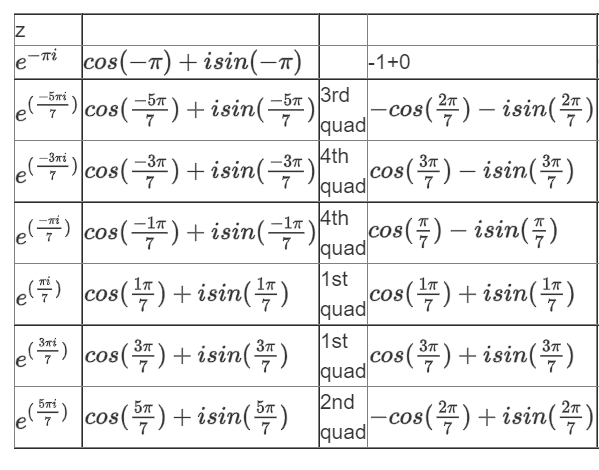
The first obvious z value is e^(pi *i) the general z solution is e^(pi*i - n*2pi/7) for n=0 to 6
As shown in the complex plane pic below.
| z | 1+z | |1+z|^2 | ||||
| \(e^{-\pi i}\) | \(cos(-\pi)+isin(-\pi)\) | -1+0 | 0 | 0 | ||
| \(e^{(\frac{-5\pi i}{7})}\) | \(cos(\frac{-5\pi}{7})+isin(\frac{-5\pi}{7}) \) | 3rd quad | \(-cos(\frac{2\pi}{7})-isin(\frac{2\pi}{7}) \) | \([1-cos(\frac{2\pi}{7})]-isin(\frac{2\pi}{7}) \) | \([1-cos(\frac{2\pi}{7})]^2+sin^2(\frac{2\pi}{7}) \) | \([1-2cos(\frac{2\pi}{7})] \) |
| \(e^{(\frac{-3\pi i}{7})}\) | \(cos(\frac{-3\pi}{7})+isin(\frac{-3\pi}{7})\) | 4th quad | \(cos(\frac{3\pi}{7})-isin(\frac{3\pi}{7})\) | \([1+cos(\frac{3\pi}{7})]-isin(\frac{3\pi}{7})\) | \([1-cos(\frac{3\pi}{7})]^2+sin^2(\frac{3\pi}{7}) \) | \([1-2cos(\frac{3\pi}{7})] \) |
| \(e^{(\frac{-\pi i}{7})}\) | \(cos(\frac{-1\pi}{7})+isin(\frac{-1\pi}{7})\) | 4th quad | \(cos(\frac{\pi}{7})-isin(\frac{\pi}{7})\) | \([1+cos(\frac{\pi}{7})]-isin(\frac{\pi}{7})\) | \([1+cos(\frac{\pi}{7})]^2+sin^2(\frac{\pi}{7})\) | \([1+2cos(\frac{\pi}{7})]\) |
| \(e^{(\frac{\pi i}{7})}\) | \(cos(\frac{1\pi}{7})+isin(\frac{1\pi}{7})\) | 1st quad | \(cos(\frac{1\pi}{7})+isin(\frac{1\pi}{7})\) | \([1+cos(\frac{\pi}{7})]-isin(\frac{\pi}{7})\) | \([1+cos(\frac{3\pi}{7})]^2+sin^2(\frac{3\pi}{7})\) | \([1+2cos(\frac{3\pi}{7})]\) |
| \(e^{(\frac{3\pi i}{7})}\) | \(cos(\frac{3\pi}{7})+isin(\frac{3\pi}{7})\) | 1st quad | \(cos(\frac{3\pi}{7})+isin(\frac{3\pi}{7})\) | \([1+cos(\frac{3\pi}{7})]+isin(\frac{3\pi}{7})\) | \([1+cos(\frac{3\pi}{7})]^2+sin^2(\frac{3\pi}{7})\) | \([1+2cos(\frac{3\pi}{7})]\) |
| \(e^{(\frac{5\pi i}{7})}\) | \(cos(\frac{5\pi}{7})+isin(\frac{5\pi}{7})\) | 2nd quad | \(-cos(\frac{2\pi}{7})+isin(\frac{2\pi}{7})\) | \([1-cos(\frac{2\pi}{7})]+isin(\frac{2\pi}{7})\) | \([1-cos(\frac{2\pi}{7})]^2+sin^2(\frac{2\pi}{7})\) | \([1-2cos(\frac{2\pi}{7})]\) |
I'm saving now so that I don't lose it. But I am still going. I am sure this is the most rediculous way anyone has ever done it ://