AD = sqrt ( AE^2 + DE^2) =sqrt (7^2 + 24^2) = sqrt (625) = 25 = BC
FC = sqrt ( BC^2 - FB^2) =sqrt (25^2 - 15^2) =sqrt ( 625 -225) = sqrt (400) = 20
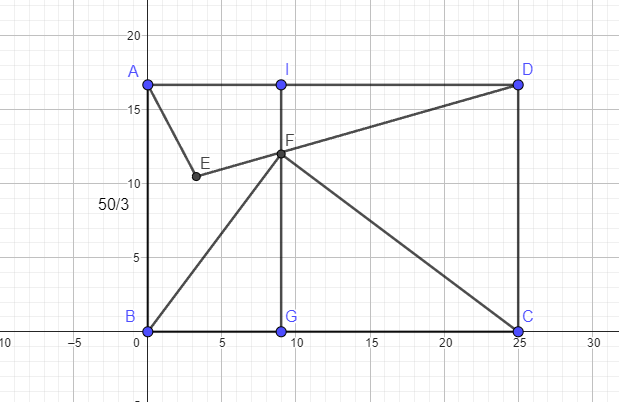
In triangle BFC draw altitude FG
Let GC = x Let BG = 25 -x
FG^2 = FB^2 - BG^2 = FB^2 - (25-x)^2 = 15^2 - (25 -x)^2
FG^2 = FC^2 - CG^2 = FC^2 - x^2 = 20^2 - x ^2
So
FG^2 = FG^2
15^2 - (25-x)^2 = 20^2 - x^2
225 - 625 + 50x - x^2 = 400 - x^2
50x = 800
x = 16 = CG
So FG = sqrt (FC^2 - CG^2) = sqrt ( 20^2 - 16^2) = sqrt (400 - 256) = sqrt (144) = 12
Extend FG through F and let it intersect AD at I
Triangle IDF is a right triangle with angle DIF = 90
Note that DI = CG
tan angle ADE = AE /DE = 7/24
tan angle ADE = tan angle IDF = FI /DI = FI /16
So
FI /16 = 7/24
FI = (7/24) (16) = (2/3) (7) = 14/3
AB = FG + FI = 12 + 14/3 = 50 / 3


