CPhill

y  = sin  ( x - 2pi)

This the sine graph  shifted to  the right by  2pi rads......however.....since the normal peiod is 2pi, it will not appear different from the normal sine graph

Here's the graph  :  https://www.desmos.com/calculator/z4edgadelv

y  = 2cos (x/4 + pi/2)

Write this as :

y  = 2cos(1/4) (x - 2pi)

This is the normal cosine graph with a period 4 times as long as normal, vertically stretched by a factor of 2  and with a phase shift of 2pi rads to the right

Here's  the parent graph and this one  on the same axis

https://www.desmos.com/calculator/u4qjyniuu7

h  = -16t^2  +  72t

The time that the ball is at the max height can be found as

-72 / [ 2 (-16) ]  =  -72 / -32  =  9/4 sec  = 2.25 sec

And the  max height is

-16(9/4)^2   +  72 (9/4)   =   81 ft

The ball wil fall back to the ground  at  2(9/4)  =  18/4  = 9/2 sec = 4.5 sec

So.....the domain   is  from 0 seconds to 4.5 seconds

And the range is from  0 ft  to   81 ft

Hre's a graph  :   https://www.desmos.com/calculator/i1dbl46fd9

1. 

a)  It makes one revolution every 3 seconds....so....it makes 20 revolutions every minute

b)  The linear velocity  is  2pi  (5) ( 120 /360)  =   (10/3)pi  inches per second

This is an isosceles trapezoid...angle  DEB   = 45° 

We can find the height, H,  of the tapezoid as

sin(45)  =  H / 12

12 sin (45)  = H

6√2   cm = H

And the longer base length is [12 + 6√2  + 6√2]  =   [ 12  + 12√2  ] cm

So....the area  is

height  *  (sum of base lengths)  / 2 =

6√2  [  12 + (12 +12√2) ] / 2  =

6√2 [ 24  + 12√2 ] / 2  =

3√2  [ 24  + 12√2]  =

72√2  + 72  =

72 (√2 + 1)  cm^2  

Angles A  and C  are supplementary     And B and D  are supplementary [sum to 180 degrees ]

So we have

(x + 2)  + ( x - 2)  =180

2x  = 180     divide both sides by 2

x  = 90

So  A  =   90 + 2 = 92 degrees

C  =  90 - 2  =  88 degrees

D  = 90  - 10  =  80 degrees

And  B = 180  - D   =  180  - 80  = 100 degrees

In the form  y  =  mx + b.....m is the slope and b is the y intercept

The slope is negative....so....we can automaticaly eliminate A  and B

Note that the y intercept is positive

The only answer that works is  "C"

Second one

There is no horizontal asymptote here....this will only happen when the polynomials in the numerator and denominator have the same degree

LOL!!!

Here's what EP  is saying

Volume  =  (4/3)pi (radius)^3   = (4/3)pi (5)^3   =  (4/3)pi* 125 ≈ 523.6  units^3 

Surface Area =  4pi (radius)^2  =  4pi (5)^2  = 100pi  ≈  314.16 units^2

We can find the height of the ttrapezoid thusly

300  =  h ( 30 + 20) /2

300 = h * 25

!2  = h

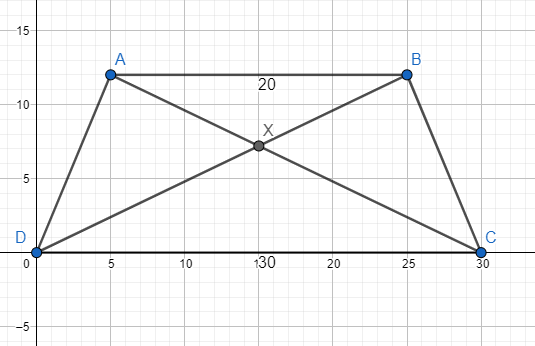
Let A  = (5,12)   B  = (25,12)  C  = (30,0)  and D  = (0,0)

The area  of triangle DBC  = (1/2) CD * 12  =  (1/2) (30) * 12  =  180  units^2     (1)

The  equation  of line segment BD  is

y  =(12/25)x

The y coordinate  of X   is the height of triangle CXD and   can be found as

y  =  (12/25) (15)   = 3/5 * 12  = 7.2

So....the area of triangle  CXD  = (1/2)(CD)(7.2)  = (1/2)(30)(7.2)  = 108 units^2     (2)

So...the area  of triangle BXC  =  (1)  - (2)  =  180  - 108  =  72 units^2

Here's a pic :