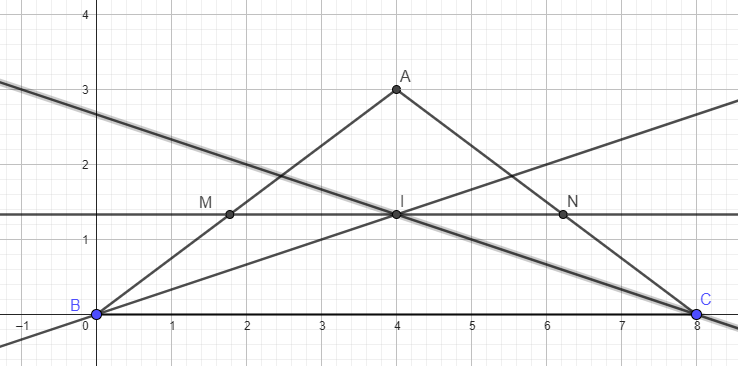
Let B = (0,0)
C =(8,0)
Construct a circle with a radius of 5 centered at B
The equation is x^2 + y^2 = 25
Since AB = AC, the triangle is isosceles and A = (BC/2, y) = (8/2, y) = (4,y)
The x coordinate of A = 4
To find y
4^2 + y^2 =25
16 + y^2 = 25
y^2 = 9
y = 3 = the height of ABC
So A = (4,3)
The incenter will occur at (4, m)
To find m
[ 5*0 + 5*0+ 8*3 ] /[5 + 5 + 8] = 24 / 18 = 4/3
So I = (4, 4/3)
Triangles ABC and AMN are similar
[ABC] = (1/2)(BC)(height) = (1/2) (8) (3) = 12
Height of AMN = 3 - 4/3 = 5/3
Scale factor of AMN to ABC = (5/3) / 3 = 5/9
Area of AMN = Area of ABC * (5/9)^2 = 12 (5/9)^2 = 100 / 27


